Shift-omefel's Theorem

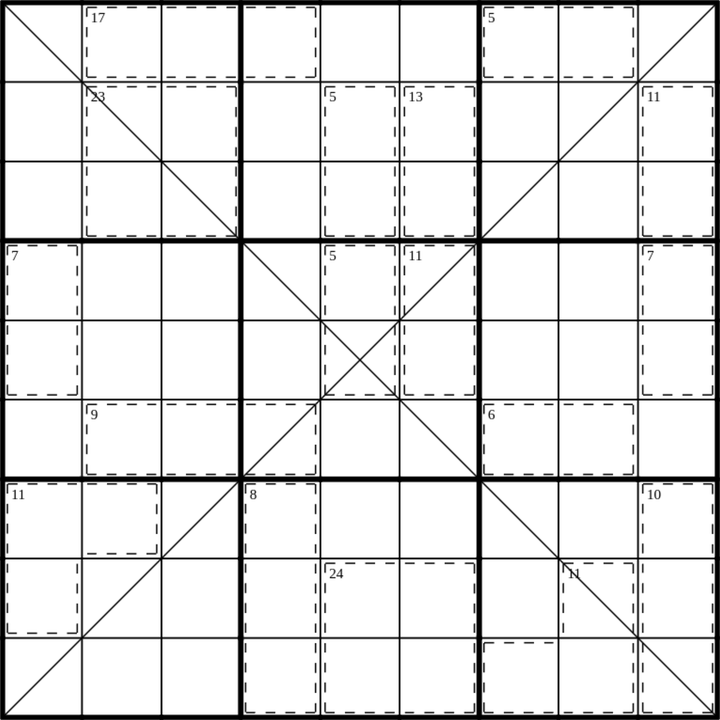
If you highlight rows, columns, and boxes of the puzzle in two equally sized sets those sets will contain the same digits. Any cells that are in both sets can be removed without affecting this equivalence. This idea is known as “set.” From this simple idea, you can find that the digits in the 2x2 squares which appear at the far corners of the puzzle are equivalent to the 16 cells which ring the central box. This is Phistomefe’s Theorem and was a relatively new discovery at the time this puzzle was published. This puzzle is made easier (in my opinion) by using set.

Ordinary Sudoku Rules Apply

Digits may not repeat on each of the main diagonals

Ordinary Partial Killer Rules Apply

try it here